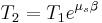Belt friction
Belt friction is a term describing the friction forces between a belt and a surface, such as a belt wrapped around a bollard. When one end of the belt is being pulled only part of this force is transmitted to the other end. The friction force makes that the tension in the belt can be different at both ends of the belt. Belt friction can be modeled by the Belt friction equation.[1]>
In practice, the theoretical tension acting on the belt or rope calculated by the belt friction equation can be compared to the maximum tension the belt can support. This helps a designer of such a rig to know how many times the belt or rope must be wrapped around the pulley to prevent it from slipping. Mountain climbers and sailing crews demonstrate a standard knowledge of belt friction when accomplishing basic tasks.
Contents |
Equation
The equation used to model belt friction is, assuming the belt has no mass and its material is a fixed composition:[2]
where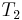 
is the tension of the pulling side, which is typically the greater force, 
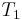is the tension of the resisting side, 
is the static friction coefficient, which has no units, and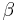 
is the angle, in radians formed by the first and last spots the belt touches the pulley, with the vertex at the center of the pulley.[3]
The tension on the pulling side has the ability to increase exponentially[1] if the size of the angle increases (e.g. it is wrapped around the pulley segment numerous times) and as the coefficient of friction grows.
Friction coefficient
There are certain factors that help determine the value of the friction coefficient. These determining factors are:[4]
- Belting material used – The age of the material also plays a part, where worn out and older material tends to be more rough and therefore experience greater friction when sliding.
- Construction of the drive-pulley system – This involves strength and stability of the material used, like the pulley, and how greatly it will oppose the motion of the belt or rope.
- Conditions in which the test is being – The friction between the belt and pulley will decrease immensely if the belt happens to be muddy or wet, as it acts as lubricant to the force. This also applies to extremely dry or warm conditions which will evaporate any water naturally found in the belt, making friction much greater.
- Overall design of the setup – The setup involves the initial conditions of the construction, such as the angle which the belt is wrapped around and the maximum amount of tension that can be sustained by the belt.
Applications
An understanding of belt friction is essential for sailing crews and mountain climbers.[1] Their professions require being able to maximize the amount of weight a rope with a certain tension capacity can hold versus the amount of wraps around a pulley. Too many revolutions around a pulley make it inefficient to retract or release rope, and too few may cause the rope to slip. Misjudging the ability of a rope to sustain itself against a certain force will ultimately lead to failure or serious injury.
See also
References
- ^ a b c Attaway, Stephen W. (1999). "The Mechanics of Friction in Rope Rescue" (PDF). International Technical Rescue Symposium. http://www.jrre.org/att_frict.pdf. Retrieved February 1, 2010.
- ^ Mann, Herman (May 5, 2005). "Belt Friction". Ruhr-Universität. http://www.esr.ruhr-uni-bochum.de/rt1/currentcourse/node57.html. Retrieved 2010-02-01.
- ^ Chandoo. "Couloumb Belt Friction". Missouri University of Science and Technology. http://web.mst.edu/~bestmech/preview/chandoo/8_2_2_1/8_2_2_1.htm. Retrieved 2010-02-01.
- ^ "Belt Tension Theory". CKIT – The Bulk Materials Handling Knowledge Base. http://www.ckit.co.za/secure/conveyor/troughed/belt_tension/belt_tension_factors.htm. Retrieved 2010-02-01.